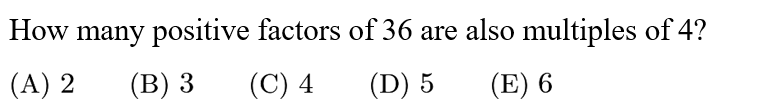
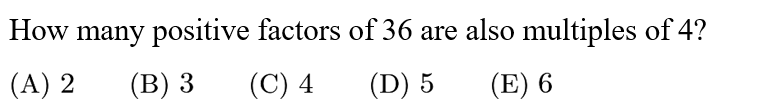
Answer: B
Solution:
The factors of 36 are: 1, 2, 3, 4, 5, 9, 12, 18, 36. Which 3, 12, 36 are multiples of 4.

Answer: C
Solution:
Jose: 10-1=9, 9*2=18, 18+2=20;
Thuy: 10*2=20, 20-1=19, 19+2=21;
Kareem: 10-1=9, 9+2=11, 11*2=22. The largest one is Kareem’s.
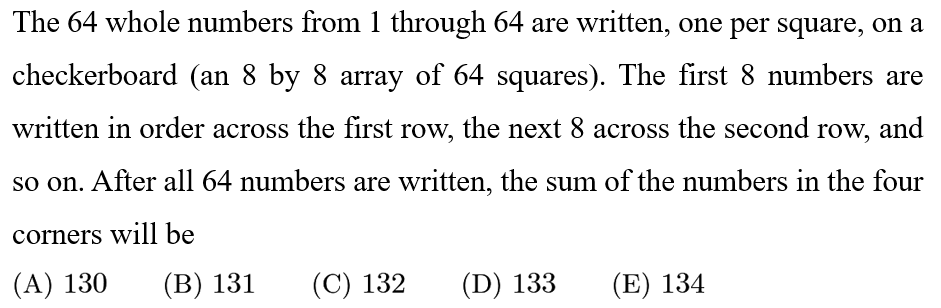
Answer: A
Solution:
Obviously 1 is in the top left corner, 8 is in the top right corner, and 64 is in the bottom right corner. To find the bottom left corner, subtract 7 from 64 which is 57. Adding the results gives 1+8+57+64=130 which is answer A.
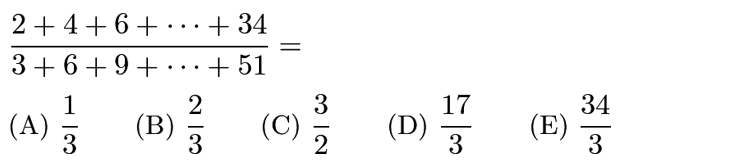
Answer: B
Solution:
Obviously 1 is in the top left corner, 8 is in the top right corner, and 64 is in the bottom right corner. To find the bottom left corner, subtract 7 from 64 which is 57. Adding the results gives 1+8+57+64=130 which is answer A.
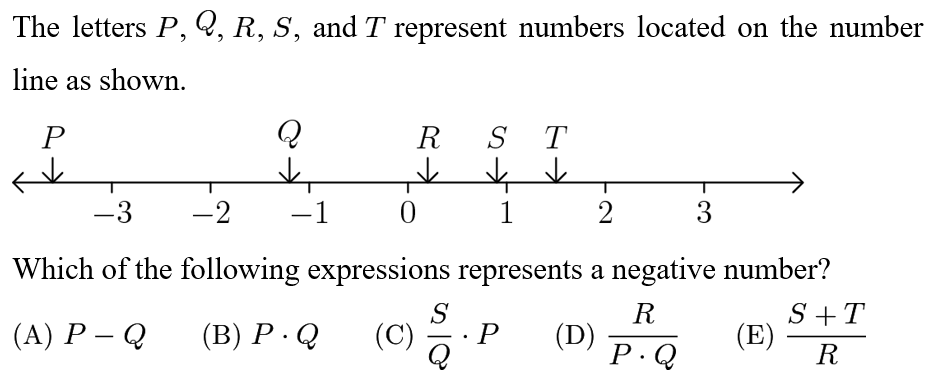
Answer: A
Solution:
Option A: P<0, Q<0, and |P|>|Q|, so P-Q=-|p|+|Q|=|Q|-|P|<0;
Option B : P and Q are all negative number ,so P*Q >0;
Option C: P<0, Q<0, => P*Q>0; S>0; => S/Q*P >0;
Option D: P<0, Q<0, =>P*Q>0;=>1/(P*Q)>0=>R/(P*Q)>0;
Option E: S>0, T>0 => S+T>0; => (S+T)/R >0.

Answer: C
Solution:
Since we want the smallest possible result, and we are only adding and multiplying positive numbers over 1, we can "prune" the set to the three smallest numbers {3,5,7}. Add the two smaller, then multiply with the biggest one. (5+7)*3=36.

Answer: B
Solution:
Month 1: Brent: 4*4, Cretel: 128*2;
Month 2: Brent: 4*4^2; Cretel: 128*2^2
…
Month n: Brent: 4*4^n; Cretel: 128*2^n
Let 4*4^n=128*2^2 =>n=5.

Answer: B
Solution:
when ABCD are all on a line, the AD are closest. AD=10-4-3=3.
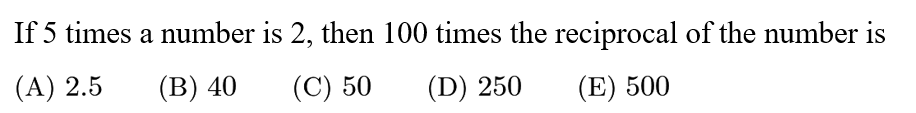
Answer: D
Solution:
5*x=2 => x=2/5; 100*5/2=250.

Answer: D
Solution:
The tank started at 1/8 full, and ended at 5/8 full. Therefore, Walter filled 5/8-1/8=1/2 of the tank. If Walter fills half the tank with 7.5 gallons, then Walter can fill two halves of the tank (or a whole tank) with 7.5*2=15 gallons, giving an answer of D.

Answer: D
Solution:
stimate each of the options.
A will give a number that is just over 3.
B will give a number that is just under 3.
C will give a number that is barely over 0, since it is three times a tiny number.
D will give a huge number. 1/x will get very, very large in magnitude when x gets close to zero.
E will give a small number, since you're dividing a tiny number into thirds.
The largest number is D.

Answer: B
Solution:
1+2+3+…+11=66. Since there are 11 numbers on the list, taking 1 number away will leave 10 numbers. If those 10 numbers have an average of 6.1, then those 10 numbers must have a sum of 10*6.1=61. Thus, the number that was removed must be 66-61=5, and the answer is B.

Answer: E
Solution:
1996: 800; 1997: 800*1.5; 1998: 800*1.5^2; 1999: 800*1.5^3=2700.
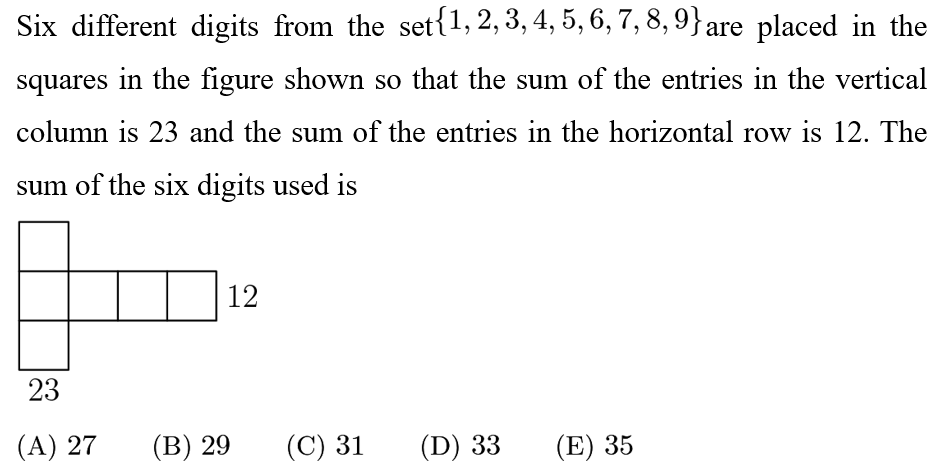
Answer: B
Solution:
Looking at the vertical column, the three numbers sum to 23. If we make the numbers on either end 9 and 8 in some order, the middle number will be 6. This is the minimum for the intersection.
Looking at the horizontal row, the four numbers sum to 12. If we minimize the three numbers on the right to 1,2,3, the first number has a maximum value of 6. This is the maximum for the intersection
Thus, the minimum of the intersection is 6, and the maximum of the intersection is 6. This means the intersection must be 6, and the other numbers must be 9 and 8 in the column, and 1,2,3 in the row. The sum of all the numbers is 12+23-6=29.

Answer: E
Solution:
To determine a remainder when a number is divided by 5, you only need to look at the last digit. To determine the last digit of 1492*1776*1812*1996, you only need to look at the last digit of each number in the product. Thus, we compute 2*6*2*6=144. The last digit of the number 1492*1776*1812*1996 is also 4, and thus the remainder when the number is divided by 5 is also 4.
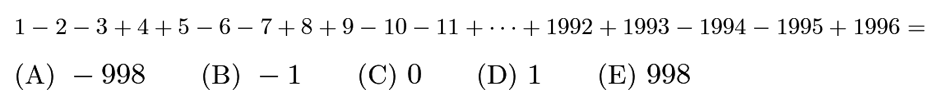
Answer: C
Solution:
=(1-2-3+4)+(5-6-7+8)+…+(1993-1994-1995+1996)=0+0+…+0=0.
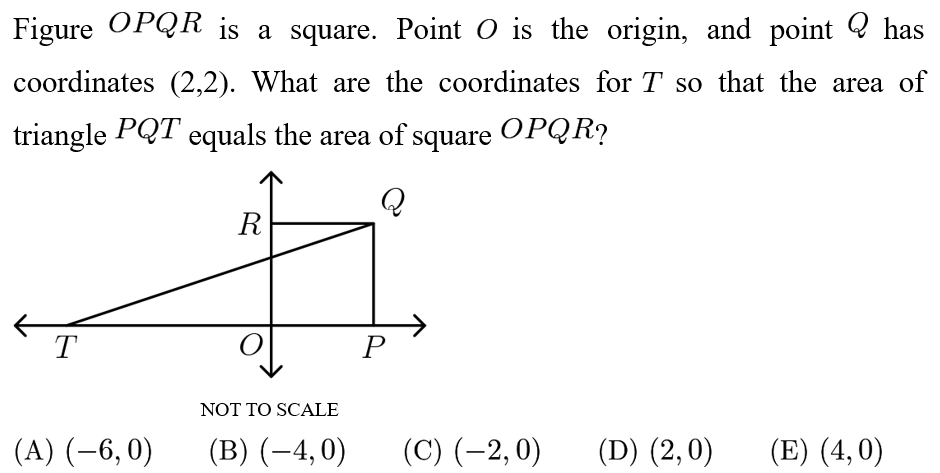
Answer: C
Solution:
Answer: A
Solution:
In June, Ana's pay is 2000*1.2=2400; In July, Ana's pay is 2400*0.8=1920.

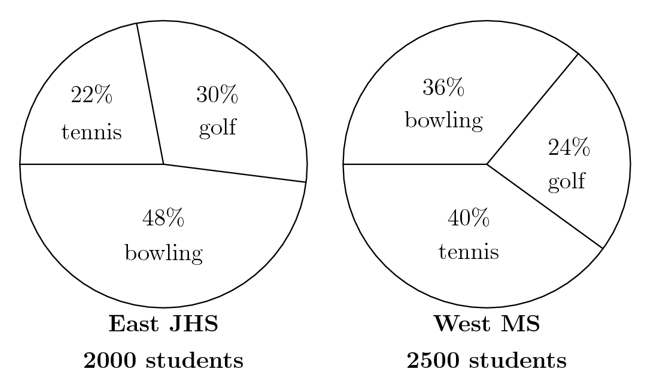
Answer: C
Solution:
(22%*2000+40%*2500)/(2000+2500)=32%
Answer: A
Solution:
We look for a pattern, hoping this sequence either settles down to one number, or that it forms a cycle that repeats.
After 1 press, the calculator displays 1/(1-5)=-1/4;
After 2 presses, the calculator displays 1/(1- -1/4)=4/5;
After 3 presses, the calculator displays 1/(1-4/5)=5;
Thus, every three presses, the display will be 5. On press 3*33=99,the display will be 5. One more press will give -1/4. Which is answer A.

Answer: D
Solution:
To have an even sum with three numbers, we must add either E+O+O,or E+E+E(no 3 even number in this data set). There are 4 choices for the first odd number and 3 choices for the last odd number. But the order of picking these numbers doesn't matter, so this overcounts the pairs of odd numbers by a factor of 2. Thus, we have (4*3)/2=6 choices for a pair of odd numbers. In total, there are 2 choices for an even number, and 6 choices for the odd numbers, giving a total of 2*6=12 possible choice.
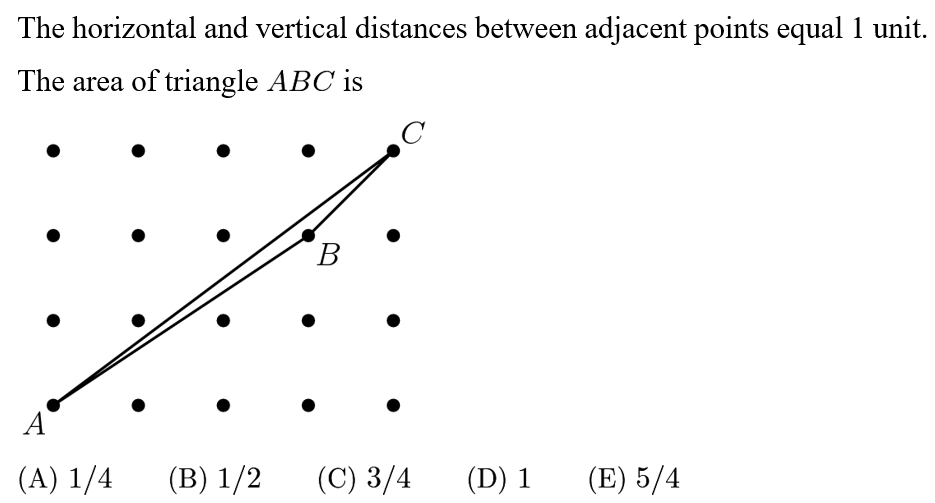
Answer: B
Solution:
Let the right bottom point is D; BF perpendicular with AD at F. BEperpendicular with CD at E.
Area(ABC)=Area(ACD)-Area(ABF)-Area(BEDF)-Area(BCE)=1/2*4*3-1/2*3*2-1*2-1/2*1*1=1/2.

Answer: E
Solution:
Let p be the number of people in the company, and f be the amount of money in the fund.
The first sentence states that 50p=f+5;
The second sentence states that 45p=f-95.
Subtracing the second equation from the first, we get 5p=100, leading to p=20.
Plugging that number into the first equation gives 50*20=f+5, leading to f=995, which is answer E.
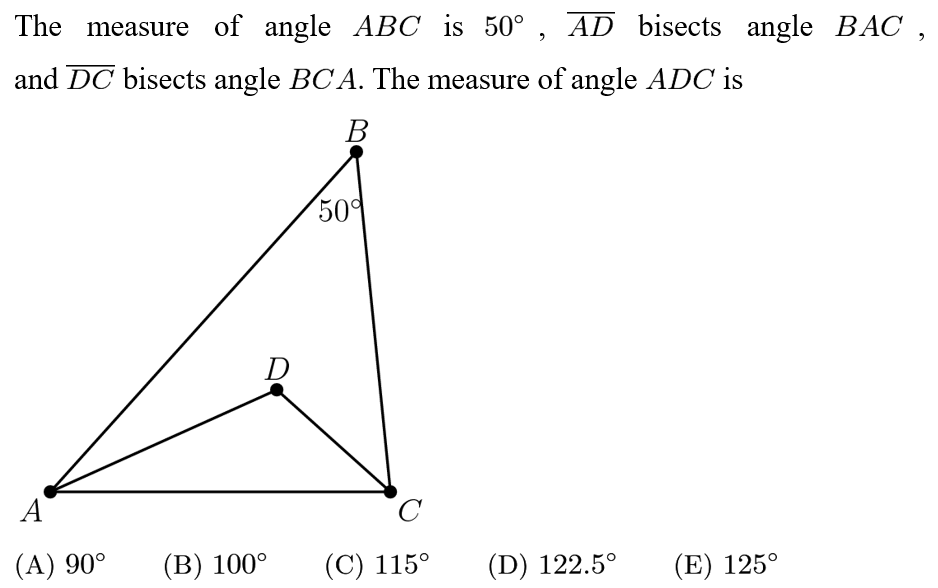
Answer: C
Solution:
Let <CAD=<BAD=x, let <ACD=<BCD=y.
From triangle ABC, we know that 50+2x+2y=180, leading to x+y=65.
From triangle ADC, we know that x+y+<D=180, Plugging in x+y=65, we get <D=180-65=115.

Answer: A
Solution:
AMC培训、答疑,请联系微信 / 电话:136 1118 1627
艾蕾特教育 @Elite Edu, 在这里,